算法
算法
查找算法
基本查找
也叫顺序查找
说明:顺序查找适合存储结构为数组或者链表
基本思想:
顺序查找也称为线形查找,属于无序查找算法。从数据结构线的一端开始,顺序扫描,依次将遍历到的结点与要查找的值相比较,若相等则表示查找成功;若遍历结束仍没有找到相同的,表示查找失败。
1 | |
二分查找
也叫折半查找
说明:
元素必须是有序的,从小到大或者从大到小都是可以的 如果是无序的,也可以先进行排序。但是排序之后,会改变原有数据的顺序,查找出来元素位置跟原来的元素可能是不一样的,所以排序之后再查找只能判断当前数据是否在容器当中,返回的索引无实际的意义。
基本思想:
也称为是折半查找,属于有序查找算法。给定值先与中间节点比较。比较完之后有三种情况
- 相等:说明找到了
- 要查找的数据比中间节点小:说明要查找的数字在中间节点的左边
- 要查找的数据比中间节点大:说明要查找的数字在中间节点的右边
1 | |
插值查找
在介绍插值查找之前,先考虑一个问题:
为什么二分查找算法一定要是折半,而不是折四分之一或者折更多呢?
其实就是因为方便,简单,但是如果我能在二分查找的基础上,让中间的mid点,尽可能靠近想要查找的元素,那不就能提高查找的效率了吗?
二分查找中查找点计算如下:
mid=(low+high)/2, 即mid=low+1/2*(high-low);
我们可以将查找的点改进为如下:
mid=low+(key-a[low])/(a[high]-a[low])*(high-low),
这样,让mid值的变化更靠近关键字key,这样也就间接地减少了比较次数。
基本思想:
基于二分查找算法,将查找点的选择改进为自适应选择,可以提高查找效率。当然,插值查找也属于有序查找。细节:对于表长较大,而关键字分布又比较均匀的查找表来说,插值查找算法的平均性能比折半查找要好的多。反之,数组中如果分布非常不均匀,那么插值查找未必是很合适的选择。
代码跟二分查找类似,只要修改一下mid的计算方式即可。
1 | |
斐波那契查找
在介绍斐波那契查找算法之前,我们先介绍一下很它紧密相连并且大家都熟知的一个概念——黄金分割。
黄金比例又称黄金分割,是指事物各部分间一定的数学比例关系,即将整体一分为二,较大部分与较小部分之比等于整体与较大部分之比,其比值约为1:0.618或1.618:1。
0.618被公认为最具有审美意义的比例数字,这个数值的作用不仅仅体现在诸如绘画、雕塑、音乐、建筑等艺术领域,而且在管理、工程设计等方面也有着不可忽视的作用。因此被称为黄金分割。
在数学中有一个非常有名的数学规律:斐波那契数列:1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89…….
(从第三个数开始,后边每一个数都是前两个数的和)。
然后我们会发现,随着斐波那契数列的递增,前后两个数的比值会越来越接近0.618,利用这个特性,我们就可以将黄金比例运用到查找技术中。
基本思想:也是二分查找的一种提升算法,通过运用黄金比例的概念在数列中选择查找点进行查找,提高查找效率。同样地,斐波那契查找也属于一种有序查找算法。
斐波那契查找也是在二分查找的基础上进行了优化,优化中间点mid的计算方式即可
1 | |
分块查找
当数据表中的数据元素很多时,可以采用分块查找。汲取了顺序查找和折半查找各自的优点,既有动态结构,又适于快速查找
分块查找适用于数据较多,但是数据不会发生变化的情况,如果需要一边添加一边查找,建议使用哈希查找
分块查找的过程:
- 需要把数据分成N多小块,块与块之间不能有数据重复的交集。
- 给每一块创建对象单独存储到数组当中
- 查找数据的时候,先在数组查,当前数据属于哪一块
- 再到这一块中顺序查找
1 | |
哈希查找
哈希查找是分块查找的进阶版,适用于数据一边添加一边查找的情况。
一般是数组 + 链表的结合体或者是数组+链表 + 红黑树的结合体
为了让大家方便理解,所以规定:
- 数组的0索引处存储1~100
- 数组的1索引处存储101~200
- 数组的2索引处存储201~300
- 以此类推
但是实际上,我们一般不会采取这种方式,因为这种方式容易导致一块区域添加的元素过多,导致效率偏低。
更多的是先计算出当前数据的哈希值,用哈希值跟数组的长度进行计算,计算出应存入的位置,再挂在数组的后面形成链表,如果挂的元素太多而且数组长度过长,我们也会把链表转化为红黑树,进一步提高效率。

数表查找
基本思想:二叉查找树是先对待查找的数据进行生成树,确保树的左分支的值小于右分支的值,然后在就行和每个节点的父节点比较大小,查找最适合的范围。 这个算法的查找效率很高,但是如果使用这种查找方法要首先创建树。
二叉查找树(BinarySearch Tree,也叫二叉搜索树,或称二叉排序树Binary Sort Tree),具有下列性质的二叉树:
1)若任意节点左子树上所有的数据,均小于本身;
2)若任意节点右子树上所有的数据,均大于本身;
二叉查找树性质:对二叉查找树进行中序遍历,即可得到有序的数列。
不同形态的二叉查找树如下图所示:
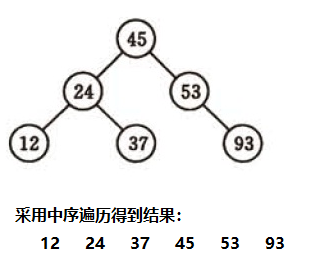
基于二叉查找树进行优化,进而可以得到其他的树表查找算法,如平衡树、红黑树等高效算法。
不管是二叉查找树,还是平衡二叉树,还是红黑树,查找的性能都比较高
排序算法
冒泡排序
冒泡排序(Bubble Sort)也是一种简单直观的排序算法。
它重复的遍历过要排序的数列,一次比较相邻的两个元素,如果他们的顺序错误就把他们交换过来。
这个算法的名字由来是因为越大的元素会经由交换慢慢”浮”到最后面。当然,大家可以按照从大到小的方式进行排列。
算法步骤
- 相邻的元素两两比较,大的放右边,小的放左边
- 第一轮比较完毕之后,最大值就已经确定,第二轮可以少循环一次,后面以此类推
- 如果数组中有n个数据,总共我们只要执行n-1轮的代码就可以

1 | |
选择排序
算法步骤
- 从0索引开始,跟后面的元素一一比较
- 小的放前面,大的放后面
- 第一次循环结束后,最小的数据已经确定
- 第二次循环从1索引开始以此类推
- 第三轮循环从2索引开始以此类推
- 第四轮循环从3索引开始以此类推。
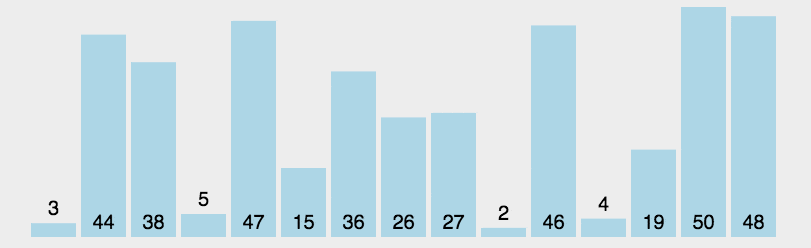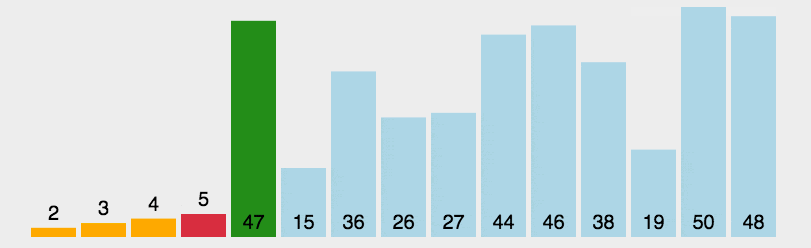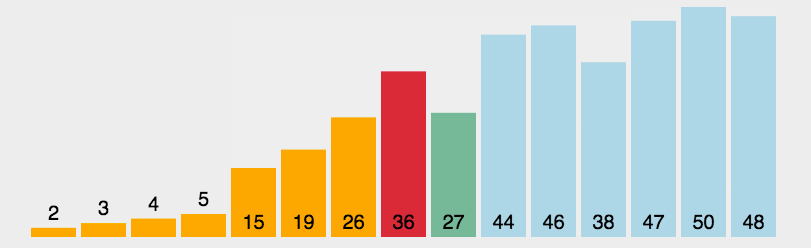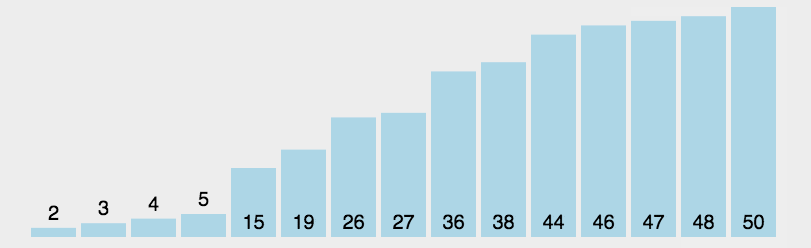
1 | |
插入排序
插入排序的代码实现虽然没有冒泡排序和选择排序那么简单粗暴,但它的原理应该是最容易理解的了,因为只要打过扑克牌的人都应该能够秒懂。插入排序是一种最简单直观的排序算法,它的工作原理是通过创建有序序列和无序序列,然后再遍历无序序列得到里面每一个数字,把每一个数字插入到有序序列中正确的位置。
插入排序在插入的时候,有优化算法,在遍历有序序列找正确位置时,可以采取二分查找
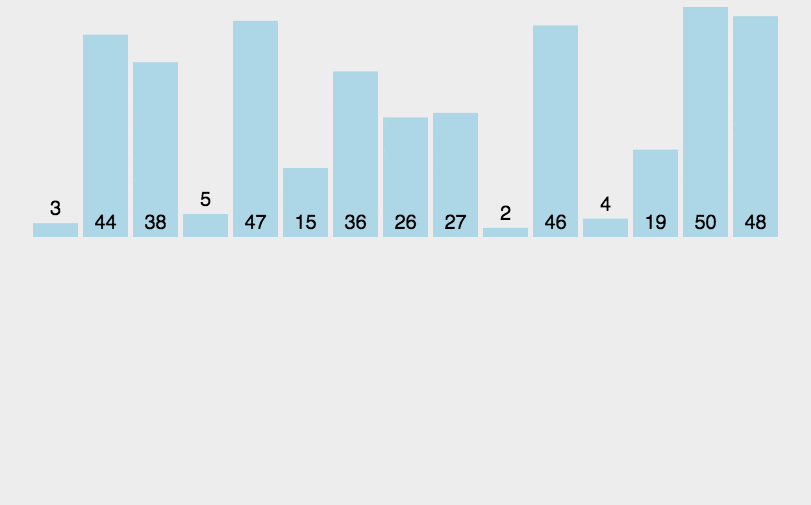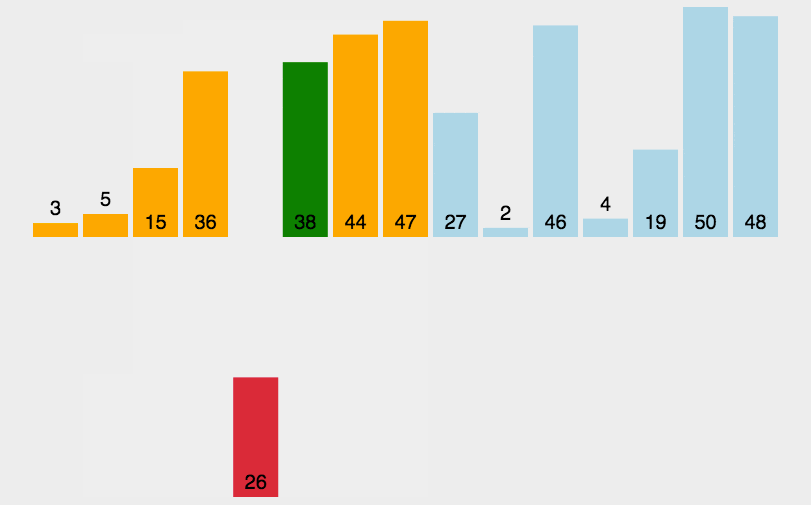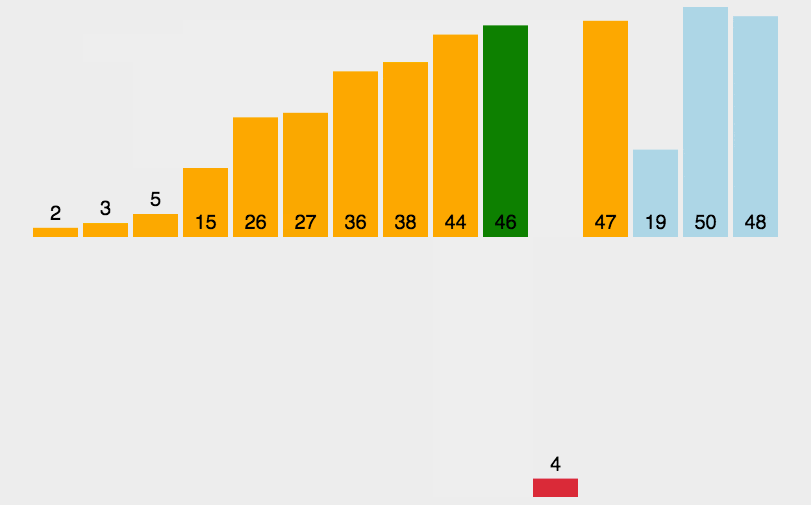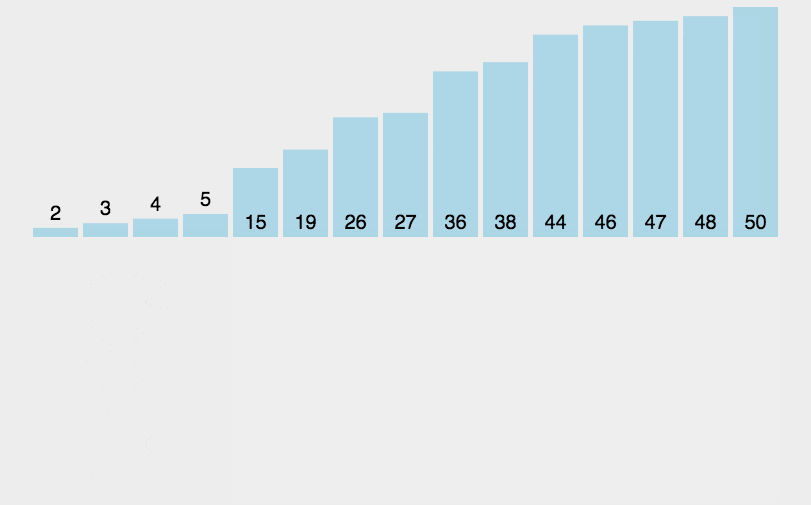
1 | |
快速排序
快速排序是由东尼·霍尔所发展的一种排序算法。
快速排序又是一种分而治之思想在排序算法上的典型应用。
快速排序的名字起的是简单粗暴,因为一听到这个名字你就知道它存在的意义,就是快,而且效率高!
它是处理大数据最快的排序算法之一了。
算法步骤
- 从数列中挑出一个元素,一般都是左边第一个数字,称为 “基准数”;
- 创建两个指针,一个从前往后走,一个从后往前走。
- 先执行后面的指针,找出第一个比基准数小的数字
- 再执行前面的指针,找出第一个比基准数大的数字
- 交换两个指针指向的数字
- 直到两个指针相遇
- 将基准数跟指针指向位置的数字交换位置,称之为:基准数归位。
- 第一轮结束之后,基准数左边的数字都是比基准数小的,基准数右边的数字都是比基准数大的。
- 把基准数左边看做一个序列,把基准数右边看做一个序列,按照刚刚的规则递归排序
1 | |
